Mathematical model for the dynamics of bacterial meningitis (Meningococcal meningitis): a case study of Yobe State Specialist Hospital, Damaturu, Nigeria

Umar Yusuf Madaki; Adamu Shu’aibu; Muhammad Idris Umar

doi:10.54117/gjpas.v2i2.19

Authors

Umar Yusuf Madaki Department of Mathematics and Statistics, Faculty of Science, Yobe State University, Damaturu- Nigeria
Adamu Shu’aibu Department of Mathematics and Statistics, Faculty of Science, Yobe State University, Damaturu- Nigeria
Muhammad Idris Umar Department of Statistics, Faculty of Science, Nassarawa State University, Keffi- Nigeria

DOI:

https://doi.org/10.54117/gjpas.v2i2.19

Keywords:

Bacterial meningitis, Damaturu, Disease free equilibrium (DFE), Endemic equilibrium point (EEP), Meningococcal meningitis, Reproduction number, SCIR model

Abstract

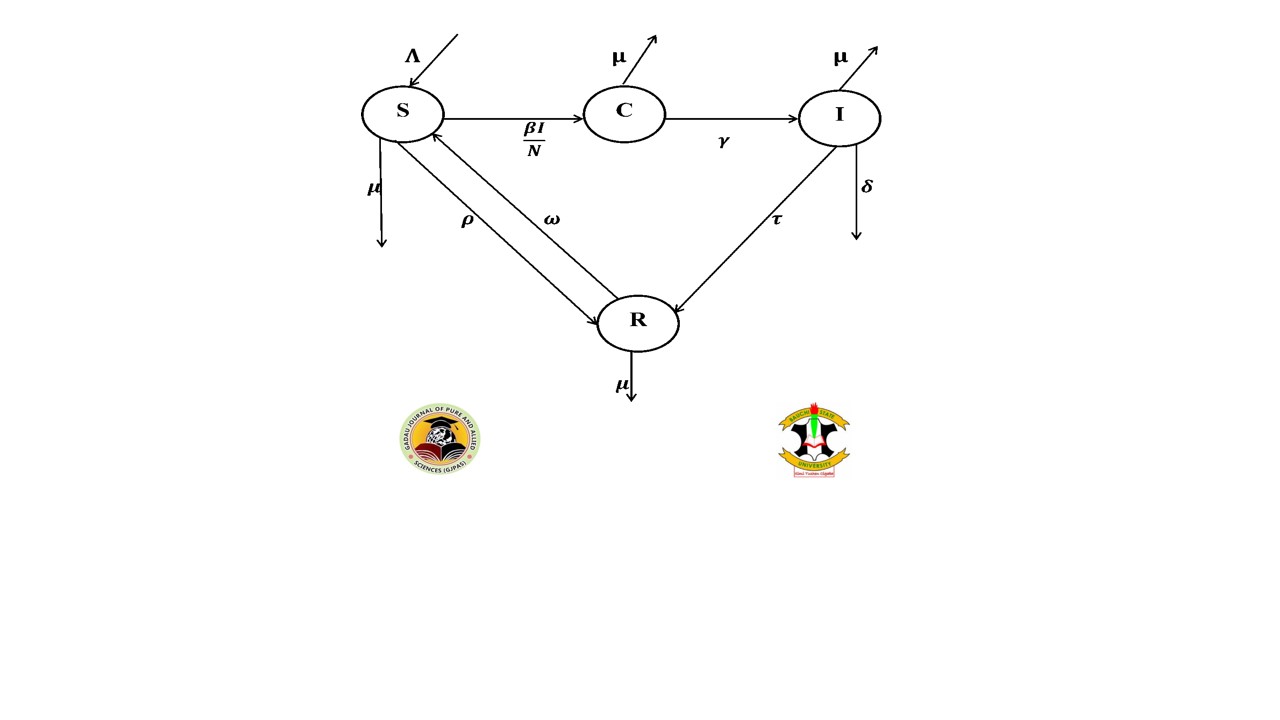
A model for bacterial meningitis was created by adding a class of transporters to the basic Susceptible Carrier Infected and Recovered (SCIR) model, since vaccination and treatment are the best methods of controlling the transmission of most overpowering sicknesses. Immunization assists helpless people with building either a drawn out invulnerability or transient resistance while treatment decreases the quantity of sickness actuated passing and the quantity of irresistible people locally or country. This study comprises of a mathematical model for bacterial meningitis dynamics that can be used to a wide range of mathematical modeling problems. In this exploration, a nonlinear deterministic model with time reliance controls has been proposed to depict the elements of bacterial meningitis in a populace. We discovered that the (EEP) and (DFE) are locally asymptotically stable in our study. We now advise the researcher to determine whether it is globally asymptotically stable in order to achieve optimal disease control. The presence of an endemic harmony and the calculation of the reproduction number . The mathematical arrangement shows that vaccinating vulnerable people will prompt disposal of the infection in the public and furthermore it will lessen the weight on wellbeing suppliers. Numerical simulations were presented to explain the parameters in the end path in the model were carried out by using MAPLE software. Most likeable of this research work shows that the rate at which treatment and vaccination rate increases to the higher value the recovered compartment increases to the peak point. This means treatment and vaccination has an impact on reducing the case of bacterial meningitis in a population. Now we conclude that a high infection transmission rate requires a high vaccine and treatment rate on the effect of vaccination against meningitis.

References

Asamoah, J. K. K., Nyabadza, F., Jin, Z., Bonyah, E., Khan, M. A., Li, M. Y., and Hayat, T. (2020). Backward bifurcation and sensitivity analysis for bacterial meningitis transmission dynamics with a nonlinear recovery rate. Chaos, Solitons and Fractals, 140, 110237.

Asamoah, J. K. K., Nyabadza, F., Seidu, B., Chand, M., and Dutta, H. (2018). Mathematical modeling of bacterial meningitis transmission dynamics with control measures. Computational and mathematical methods in medicine, 2018.

Baba, I. A., Olamilekan, L. I., Yusuf, A., and Baleanu, D. (2020). Analysis of meningitis model: A case study of northern Nigeria.

Blyuss, K. B. (2016). Mathematical modeling of the dynamics of Meningococcal meningitis in Africa. In UK Success Stories in Industrial Mathematics (pp. 221-226). Springer, Cham.o, B., d'Onofrio, A., Kassa, S. M., and Workineh, Y. H. (2020). Modeling the effects of information-dependent vaccination behavior on meningitis transmission. arXiv preprint arXiv:2005.11823.

Christensen, H., Irving, T., Koch, J., Trotter, C. L., Ultsch, B., Weidemann, F.,and Hellenbrand, W. (2016). Epidemiological impact and cost-effectiveness of universal vaccination with Bexsero® to reduce meningococcal group B disease in Germany. Vaccine, 34(29), 3412-3419.

Elmojtaba, I. M., and Adam, S. O. (2017). A mathematical model for Meningitis Disease. Red Sea University Journal of Basic and Applied Sceince, 2(2), 467-472.

Irving, T. J., Blyuss, K. B., Colijn, C., and Trotter, C. L. (2012). Modeling Meningococcal meningitis in the African meningitis belt. Epidemiology and Infection, 140(5), 897-905.

Vereen, K. (2008), An SCIR model Meningococcal meningitis. Scholarscompass.vcu.edu. page 1-33, Vol. 2(1).

Musa, S. S., Zhao, S., Hussaini, N., Habib, A. G., and He, D. (2020). Mathematical modeling and analysis of Meningococcal meningitis transmission dynamics. International Journal of Biomathematics, 13(01), 2050006.

Novak, R. T., Ronveaux, O., Bita, A. F., Aké, H. F., Lessa, F. C., Wang, X., ... and Fox, L. M. (2019). Future directions for meningitis surveillance and vaccine evaluation in the meningitis belt of sub-Saharan Africa. The Journal of infectious diseases, 220(Supplement_4), S279-S285.

Paireau, J., Chen, A., Broutin, H., Grenfell, B., and Basta, N. E. (2016). Seasonal dynamics of bacterial meningitis: a time-series analysis. The Lancet global health, 4(6), e370-e377.

Saha, S. (2020). Unearthing the Unknown Causes of Meningitis. The American Journal of Tropical Medicine and Hygiene, 103(2), 544.

Sulma, S., Toaha, S., and Kasbawati, K. (2020). Stability Analysis of Mathematical Models of the Dynamics of Spread of Meningitis with the Effects of Vaccination, Campaigns and Treatment. Jurnal Matematika, Statistika dan Komputasi, 17(1), 71-81.

World Health Organization. (2019). International coordination group on vaccine provision for epidemic meningitis: report of the annual meeting: Geneva, 18 September 2018 (No. WHO/WHE/IHM/2019.1). World Health Organization.